Quarks Explained
The Quark Meme
I came across a wonderful description of the history of Quarks on an obscure CalTech web page. It’s a great story titled 50 Years of Quarks, commissioned to commemorate Caltech’s professor Murray Gell-Mann who is credited with creating the meme of quarks.
Gell-Mann chose the name “Quark” from a reference in literature, “Three quarks for Muster Mark!” in James Joyce’s Finnegan’s Wake. Here’s the passage from Finnegan’s wake that inspired him… at least he did not choose to give his new meme the name “Bandersnatches”.
Three quarks for Muster Mark!
Sure he hasn’t got much of a bark
And sure any he has it’s all beside the mark.
But O, Wreneagle Almighty, wouldn’t un be a sky of a lark
To see that old buzzard whooping about for uns shirt in the dark
And he hunting round for uns speckled trousers around by Palmerstown Park?
Hohohoho, moulty Mark!
You’re the rummiest old rooster ever flopped out of a Noah’s ark
And you think you’re cock of the wark.
Fowls, up! Tristy’s the spry young spark
That’ll tread her and wed her and bed her and red her
Without ever winking the tail of a feather
And that’s how that chap’s going to make his money and mark!
Joyce himself, reported to have said that he wrote the book Finnegan’s Wake “to keep the critics busy for 300 years by putting the language to sleep’ and that “the only demand I make of my reader is that he should devote his whole life to reading my works.”
“I can’t understand some of my critics, like Pound or Miss Weaver, for instance. They say it’s obscure. They compare it, of course, with Ulysses. But the action of Ulysses was chiefly during the daytime, and the action of my new work takes place chiefly at night. It’s natural things should not be so clear at night, isn’t it now?”
Finnegans Wake is a linguistic feast, in which dream narratives, internal monologues and philosophical references combine with neologisms and Joycean sentence structures to form a peculiar and wonderful idiolect. (James Joyce in conversation with Richard Ellmann)
I get it now… that makes sub-atomic ecology so much more understandable.
Cal-Tech in it’s wisdom choose to give to Gell-Mann, the man who divided the charge of electrons, the chair of Robert Millikin the man whose claim to scientific greatness was that the charge of electrons was indivisible. James Joyce would have been proud of them, one and all.
But the CalTech piece does help a bit. You can read it here….
or reproduced below (if you read to the end, there is a prize.)
50 Years of Quarks – A Milestone in Physics
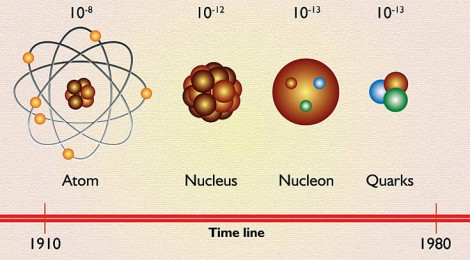
Caltech’s Murray Gell-Mann simplified the world of particle physics in 1964 by standing it on its head. He theorized that protons—subatomic particles as solid as billiard balls and as stable as the universe—were actually cobbled together from bizarre entities, dubbed “quarks,” whose properties are unlike anything seen in our world. Unlike protons, quarks cannot be separated from their fellows and studied in isolation; despite this, our understanding of the universe is built on their amply documented existence.
These days, the subatomic particle catalog has hundreds of entries. Back in the 1920s, there were only two—the massive proton, which had a charge of +1 and was found in the atom’s nucleus; and the electron, which had very little mass, a charge of –1, and orbited the nucleus. Every proton occupied one of two possible “spin states” in relation to the surrounding space. These spins could easily be flipped in a behavior described by a mathematical construct called the SU(2) symmetry group. “Quantum spin states do not have a familiar analog in everyday experience,” says Caltech’s Steven Frautschi, professor of theoretical physics, emeritus. “However, they can be turned into one another by 180-degree rotations in ordinary space, which is what SU(2) does.”
In 1932, the neutron was discovered. This new particle appeared to be the proton’s close relative—even its mass was the same, to within 0.2 percent—but the neutron had no electric charge. SU(2) symmetry in ordinary space could not account for the neutron’s existence, but quantum mechanic Werner Heisenberg fixed the problem by declaring that the two particles were indeed fraternal twins . . . if you took SU(2) from another point of view. Frautschi explains: “Like rotating a physical object in ordinary space, Heisenberg extended SU(2) by rotating the symmetry group in a ‘space’ that quantum theorists made up.”
Heisenberg gave his rotation a quantum number, now called isospin, which described the particle’s interaction with the so-called strong nuclear force. (The strong force overcomes the mutual repulsion between positively charged protons, binding them and neutrons to one another and allowing stable atomic nuclei to exist.) The mathematical treatment of isospin in Heisenberg’s theoretical space was identical to that of the proton’s spin in ordinary space, allowing neutrons to turn into protons and vice versa. In the physical world, Heisenberg’s version of SU(2) is like a slowly spinning roulette wheel after the ball has come to rest—if the white ball (a proton) could transmute itself into a black ball (a neutron) and then back again to a white ball once every revolution.
A comprehensive theory of the strong force was published three years later by Hideki Yukawa of Osaka University. Quantum-mechanical forces need particles to carry them, and Yukawa calculated that the strong-force carriers would be much more massive than electrons but not nearly as massive as protons. Soon after, in 1937, Caltech research fellow Seth Neddermeyer (PhD ’35) and Nobel laureate physics professor Carl Anderson (BS ’27, PhD ’30) stumbled upon a likely candidate: a new particle with about 200 times the electron’s mass and about one-ninth the mass of the proton.
Although it was widely assumed that Neddermeyer and Anderson had found the force-carrying particles that would prove Yukawa’s theory, the paper announcing the discovery merely described them as “higher mass states of ordinary electrons.” This proved to be the case—the new particles, now called muons, did not behave as Yukawa had predicted but instead behaved exactly like electrons. This offered the first inkling that otherwise identical particles came in multigenerational “families” of very different masses.
The search for Yukawa’s strong-force carriers did not bear fruit until 1947, when particles dubbed pions finally turned up—as did kaons, the massive second-generation members of the pion family. These kaons, however, were oddly long-lived, lasting a quadrillion times longer than expected. (“Long-lived” is relative, as the average kaon decayed into other particles in less than a millionth of a second.)
Then, in 1953, Murray Gell-Mann, then at the University of Chicago, and Kazuhiko Nishijima (also at Osaka University) independently demystified the kaons’ strange longevity by proposing yet another new quantum number to explain it. This number, imaginatively called “strangeness,” permits particles possessing it to decay—but only by shedding one strangeness unit at a time. This relatively slow process created stepwise cascades of successively less-strange particles, ultimately ending in particles whose strangeness is zero.
Unfortunately, strangeness and SU(2) did not mesh mathematically. The theorists remained at an impasse; meanwhile, the experimentalists built ever-more-powerful machines that created ever-more-massive, ever-more-exotic particles whose ever-briefer existences could only be inferred by working backward from the collections of mundane particles into which they decayed.
The mushrooming catalog of discoveries defied all attempts at organization until 1961, when Gell-Mann—who had moved to Caltech in 1955—and Israeli physicist Yuval Ne’eman independently proposed sorting particles into mini-periodic tables organized by electric charge and strangeness number. Gell-Mann dubbed his version the “Eightfold Way,” after Buddhism’s Eightfold Path to enlightenment, because the tables tended to contain eight members each.
The Eightfold Way brought physicists full circle, as it proved to be a rotating SU(3) symmetry group. Just as charge had driven the isospin axis in Heisenberg’s SU(2) symmetry, strangeness provided a second, perpendicular rotation. In other words, SU(2) spun only around the y axis, as it were, but SU(3) spun on both the x and y axes simultaneously. It was as if the roulette wheel had morphed into a globe spinning around the poles while the polar axis itself spun around two points on the equator. Relationships between particles could be represented as rotations in isospin, in strangeness, or in both.
Although the Eightfold Way solved one problem, it created another. Whereas SU(2) manifests itself through doublets—the proton-neutron dichotomy—SU(3)’s hallmark is the triplet. “Nature is likely to use this fundamental representation,” says physics professor Frautschi, “but there was no sign of triplets in the data.” Triplets could be conjured into existence, however, if the rock-solid proton could be broken apart. In that case, SU(3)’s fundamental triplet could be a menu of three hypothetical entities, each with its own unique set of quantum numbers.
If the menu choices were truly independent—much like allowing a diner to order an enchilada with all beans and no rice on the side, for example—a fundamental triplet offered enough possibilities to build every massive particle known, and then some. Intermediate-mass pions and kaons would contain two menu selections; protons, neutrons, and a slew of more massive particles would be three-item combos. “It’s all about making patterns,” Frautschi explains. “You write down sets of quantum numbers, add them up, and see what fits.”
However, the numbers refused to add up. Both the two-piece kaon and a three-piece particle called the sigma came in positive, negative, and electrically neutral versions. But if the only charges available to the triplet’s members were –1, 0, and +1, no conceivable combination of choices allowed all the other quantum numbers to come out right.
This should have been the end of the story. Robert Millikan, Caltech’s first Nobel laureate, had won his prize for showing that electric charge came only in whole-numbered units. But in 1964, Gell-Mann and George Zweig (PhD ’64) independently flew in the face of all that was known by proposing that the fundamental triplet had one member with a +2/3 charge and two members with charges of –1/3.
Gell-Mann called the members of his triplet “quarks,” after the sentence “Three quarks for Muster Mark!” in James Joyce’s Finnegan’s Wake. Everything found in the old SU(2) symmetry group could be fashioned from +2/3 “up” quarks and –1/3 “down” quarks, both of which had a strangeness number of zero. A proton was up-up-down, for example; a neutron was down-down-up. The other –1/3 quark had a quantum of strangeness; adding these “strange” quarks to the mix took care of the particles that SU(2) couldn’t handle. Since this proposal was so heretical, Gell-Mann presented quarks as no more than an expedient accounting system, writing, “It is fun to speculate about the way quarks would behave if they were physical particles of finite mass (instead of purely mathematical entities . . . ).”
Zweig, meanwhile, called his theoretical constructs “aces,” as they were put together into “deuces” and “treys” to make pions and protons. He was also less circumspect than Gell-Mann. “The results . . . seem somewhat miraculous,” Zweig wrote. “Perhaps the model is . . . a rather elaborate mnemonic device. [But] there is also the outside chance that the model is a closer approximation to nature than we may think, and that fractionally charged aces abound within us.” Sadly, Zweig’s paper met a very different fate than Gell-Mann’s. Since Zweig was working as a very junior postdoctoral fellow at CERN, the European Center for Particle Physics, all his manuscripts had to be reviewed by his superiors before publication. The senior staff considered Zweig’s ideas too outré, and his paper got sent to a file room instead of a journal. He returned to Caltech soon after, joining the faculty.
Gell-Mann went on to win the Nobel Prize for Physics in 1969—although not for the quark model per se, which was still on thin ice. (The very first experiments demonstrating that protons might contain something else had been run at the Stanford Linear Accelerator the preceding year.) Instead, he was cited “for contributions and discoveries concerning the classification of elementary particles and their interactions.”
Quarks have since been shown to be physical particles with finite masses. The up quark has been found to have about half the mass of the down, while the strange quark has been shown to be some 50 times more massive—a sure sign that it represented a second generation of quarks, just as muons had turned out to be second-generation electrons. In 1974, the other second-generation quark turned up—the “charm” quark—followed three years later by the third-generation “bottom” quark. It then took nearly two decades to find what is called the “top” quark—which, as far as we know, completes the quark family tree.
Gell-Mann was named the Robert Andrews Millikan Professor of Theoretical Physics in 1967—a fitting irony that the man who showed that fractional electric charges are necessary holds the chair named for the man who showed that electric charge is indivisible.
Gell-Mann’s paper introducing the quark was all of two pages long; what has been written about quarks since then would fill warehouses. This half-century of discoveries was celebrated at a conference in the 84-year-old Gell-Mann’s honor, hosted by Caltech’s theoretical high-energy physics group in December, 2013.
Written by Douglas Smith
– See more at: http://www.caltech.edu/content/50-years-quarks#sthash.8u4l5eYF.dpuf